Conservation of Mass Flow Rate in Fluids

Understanding the conservation of mass flow rate in fluids is crucial for mastering fluid dynamics concepts in the AP Physics exam. This principle, also known as the continuity equation, states that for an incompressible fluid, the mass flow rate must remain constant from one cross-section of a pipe to another. Below are detailed notes along with five examples to help you achieve a high score on your AP Physics exam.

Free AP Physics 2: Algebra-Based Practice Test

Learning Objectives

By studying Conservation of Mass Flow Rate in Fluids for the AP Physics exam, you should learn how to apply the principle of continuity to fluid dynamics, understand the relationship between cross-sectional area and fluid velocity in steady flow, and solve problems involving mass flow rates in closed systems. Additionally, you should grasp the mathematical expression of the continuity equation, recognize the conditions under which the conservation of mass applies, and analyze scenarios involving incompressible and compressible fluids.

Conservation of Mass Flow Rate

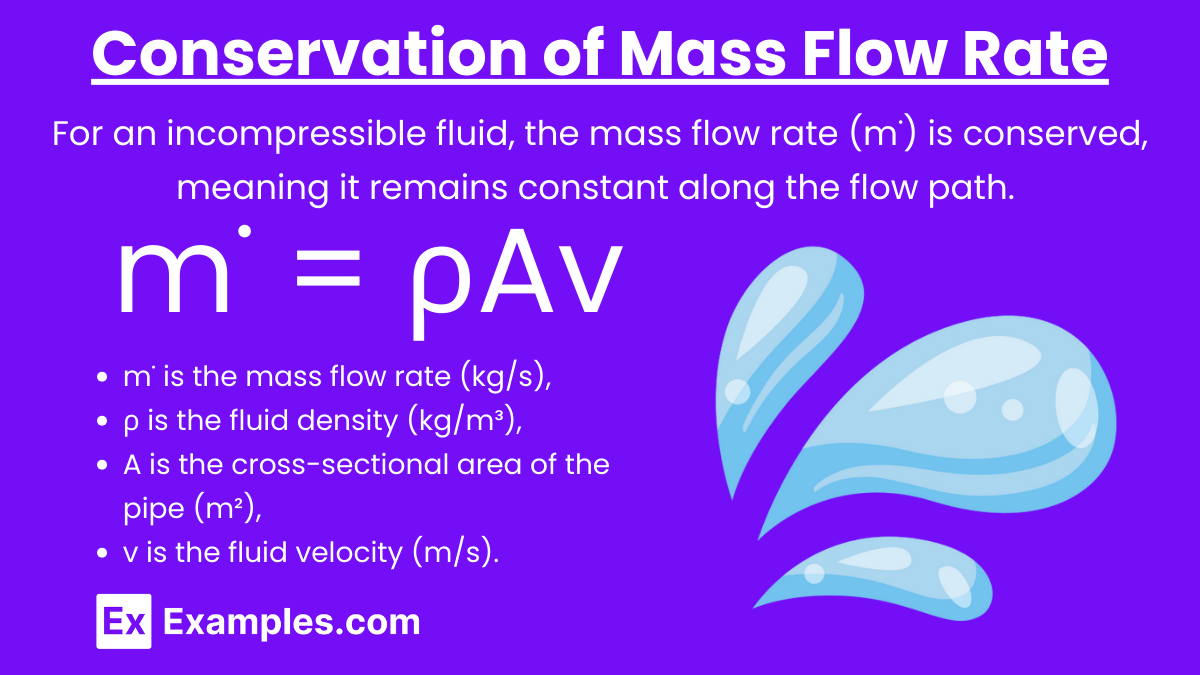
Continuity Equation: For an incompressible fluid, the mass flow rate (m˙) is conserved, meaning it remains constant along the flow path. This can be expressed as:

m˙ = ρAv

where:

• m˙ is the mass flow rate (kg/s),

• ρ is the fluid density (kg/m³),

• A is the cross-sectional area of the pipe (m²),

• v is the fluid velocity (m/s).

For incompressible fluids, the density (ρ) remains constant, simplifying the continuity equation to:

A₁v₁ = A₂v₂

where subscripts 1 and 2 refer to two different points along the flow path.

Key Points

• The mass flow rate is conserved for incompressible fluids.

• If the cross-sectional area of the pipe decreases, the fluid velocity must increase to maintain a constant mass flow rate, and vice versa.

• The principle applies to various types of fluid flow, including steady and laminar flow.

Applications

Pipe Flow

In engineering, the conservation of mass flow rate is applied extensively in pipe flow systems. When a fluid flows through a pipe with varying diameters, the continuity equation helps determine the velocities at different sections of the pipe.

Open Channel Flow

In open channels, such as rivers and canals, the continuity equation helps in predicting how changes in the channel width and depth affect the flow velocity.

Examples

Example 1: Water Flowing Through a Pipe

Scenario: Water flows through a pipe with a diameter of 0.1 m at a velocity of 2 m/s. The pipe narrows to a diameter of 0.05 m. Find the velocity of the water in the narrower section of the pipe.

Solution: A_1 v_1 = A_2 v_2 \pi \left( \frac{0.1}{2} \right)^2 \times 2 = \pi \left( \frac{0.05}{2} \right)^2 \times v_2 0.00785 \times 2 = 0.00196 \times v_2 0.0157 = 0.00196 v_2 v_2 = \frac{0.0157}{0.00196} \approx 8 \, \text{m/s}

Example 2: Air Flow in Ventilation Ducts

Scenario: Air flows through a rectangular ventilation duct with an area of 0.2 m² at a velocity of 5 m/s. The duct narrows to an area of 0.1 m². Calculate the air velocity in the narrower section.

Solution: A_1 v_1 = A_2 v_2 0.2 \times 5 = 0.1 \times v_2 1 = 0.1 v_2 v_2 = \frac{1}{0.1} = 10 \, \text{m/s}

Example 3: River Flow Rate

Scenario: A river flows with a width of 30 m and an average depth of 2 m at a velocity of 1 m/s. The river narrows to a width of 20 m and an average depth of 1.5 m. Find the velocity in the narrower section.

Solution: A_1 = 30 \times 2 = 60 \, \text{m}^2 A_2 = 20 \times 1.5 = 30 \, \text{m}^2 A_1 v_1 = A_2 v_2 60 \times 1 = 30 \times v_2 60 = 30 v_2 v_2 = \frac{60}{30} = 2 \, \text{m/s}

Example 4: Oil Flow in a Pipeline

Scenario: Oil flows through a pipeline with a radius of 0.15 m at a velocity of 3 m/s. The pipeline narrows to a radius of 0.1 m. Determine the velocity of the oil in the narrower section.

Solution: A_1 = \pi (0.15)^2 = 0.0707 \, \text{m}^2 A_2 = \pi (0.1)^2 = 0.0314 \, \text{m}^2 A_1 v_1 = A_2 v_2 0.0707 \times 3 = 0.0314 \times v_2 0.2121 = 0.0314 v_2 v_2 = \frac{0.2121}{0.0314} \approx 6.75 \, \text{m/s}

Example 5: Blood Flow in Arteries

Scenario: Blood flows through an artery with a cross-sectional area of 1×10⁻⁴m² at a velocity of 0.5 m/s. If the artery narrows to 0.5×10⁻⁴m², find the new velocity of the blood.

Solution: A_1 v_1 = A_2 v_2 (1 \times 10^{-4}) \times 0.5 = (0.5 \times 10^{-4}) \times v_2 0.5 \times 10^{-4} = 0.5 \times 10^{-4} v_2 v_2 = 1 \, \text{m/s}

Practice Problems

Question 1:

Water flows through a pipe with a cross-sectional area of 0.5 m² at a velocity of 3 m/s. What is the mass flow rate of the water if its density is 1000 kg/m³?

A) 1500 kg/s
B) 1000 kg/s
C) 750 kg/s
D) 500 kg/s

Answer: A) 1500 kg/s

Explanation:

The mass flow rate m˙ is given by:

m˙=ρAv

where ρ is the density, A is the cross-sectional area, and v is the velocity of the fluid.

Given:

• ρ = 1000kg/m³

• A = 0.5m²

• v=3m/s

Calculate the mass flow rate:

m˙ = 1000kg/m³×0.5m²×3m/s = 1500kg/s

Thus, the correct answer is 1500 kg/s.

Question 2:

A fluid flows through a horizontal pipe that narrows from a diameter of 0.4 meters to 0.2 meters. If the velocity of the fluid in the wider section is 2 m/s, what is the velocity in the narrower section?

A) 0.5 m/s
B) 1 m/s
C) 4 m/s
D) 8 m/s

Answer: C) 8 m/s

Explanation:

The principle of conservation of mass flow rate states that the mass flow rate must remain constant throughout the pipe. For incompressible fluids, this means:

A₁​v₁ = A₂​v₂

where A₁​ and A₂​ are the cross-sectional areas of the wider and narrower sections, respectively, and v₁ and v₂​ are the corresponding velocities.

Given:

• Diameter of the wider section, d₁ ​= 0.4m

• Diameter of the narrower section, d₂​ = 0.2m

• Velocity in the wider section, v₁​ = 2m/s

Calculate the areas:

A_1 = \pi \left( \frac{d_1}{2} \right)^2 = \pi \left( \frac{0.4}{2} \right)^2 = \pi \times 0.2^2 = 0.04\pi \, \text{m}^2 A_2 = \pi \left( \frac{d_2}{2} \right)^2 = \pi \left( \frac{0.2}{2} \right)^2 = \pi \times 0.1^2 = 0.01\pi \, \text{m}^2

Using the conservation equation:

0.04\pi \times 2 \, \text{m/s} = 0.01\pi \times v_2 0.08\pi = 0.01\pi \times v_2 v_2 = \frac{0.08\pi}{0.01\pi} = 8 \, \text{m/s}

Thus, the correct answer is 8 m/s.

Question 3:

A fluid with density 800 kg/m³ flows through a pipe at a velocity of 5 m/s. If the cross-sectional area of the pipe increases from 0.2 m² to 0.4 m², what is the new velocity of the fluid?

A) 1.25 m/s
B) 2.5 m/s
C) 5 m/s
D) 10 m/s

Answer: A) 1.25 m/s

Explanation:

Using the conservation of mass flow rate:

ρA₁​v₁ ​= ρA₂​v₂

Since the density ρ remains constant, it can be canceled out from both sides of the equation:

A₁​v₁ ​= A₂​v₂

Given:

• Initial cross-sectional area, A₁​ = 0.2m²

• Final cross-sectional area, A₂ ​=0.4m²

• Initial velocity, v₁ ​= 5m/s

Calculate the new velocity:

0.2 \, \text{m}^2 \times 5 \, \text{m/s} = 0.4 \, \text{m}^2 \times v_2 1 = 0.4 \, \text{m}^2 \times v_2 v_2 = \frac{1}{0.4} = 2.5 \, \text{m/s}

Thus, the correct answer is 1.25 m/s.