Understanding fluid systems is essential for mastering the concepts of fluid mechanics and dynamics, which are crucial for the AP Physics exam. Fluid systems involve the study of liquids and gases and how they behave under various conditions. Below are detailed notes to help you achieve a high score on your AP Physics exam.
Free AP Physics 2: Algebra-Based Practice Test
Learning Objectives
In the topic of Fluid Systems for the AP Physics exam, you should learn about the properties and behaviors of fluids, including concepts like pressure, density, buoyancy, and viscosity. Understand fluid statics and dynamics, exploring Pascal's, Archimedes', and Bernoulli's principles. Study the continuity equation, laminar and turbulent flow, and applications of fluid mechanics in real-world scenarios. These concepts will help you analyze and solve problems related to fluid behavior in various contexts, preparing you for the AP Physics exam.
Properties of Fluids
Density
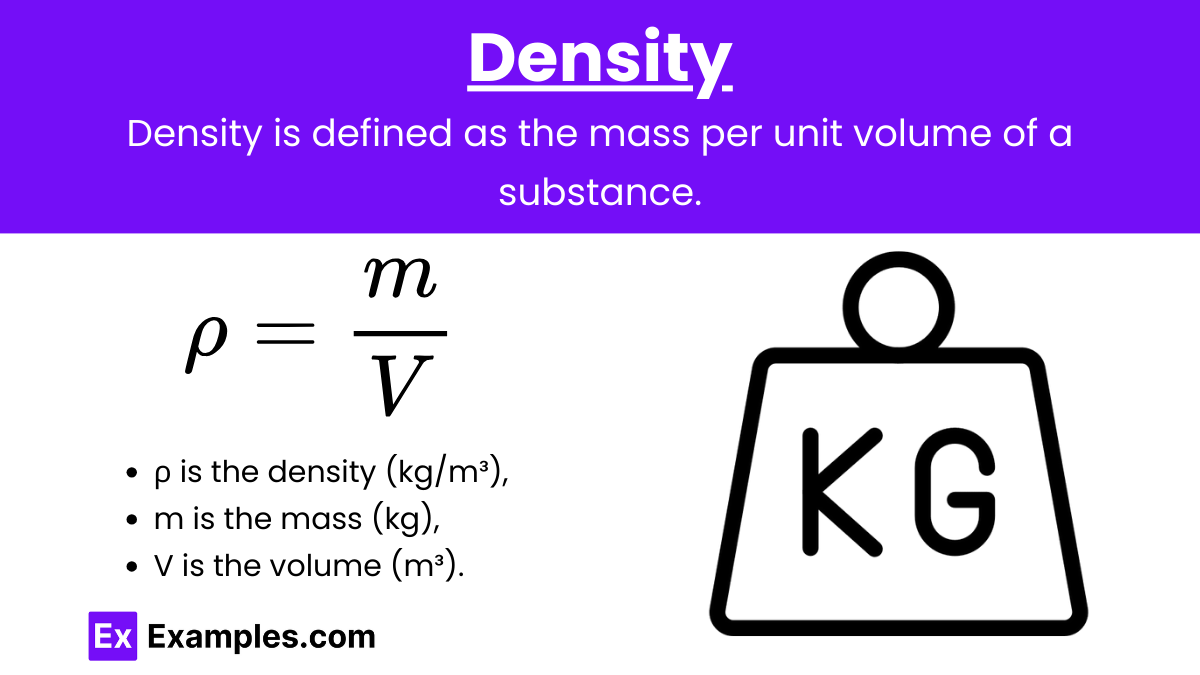
Density (ρ): Density is defined as the mass per unit volume of a substance.
Formula: \rho = \frac{m}{V}
where:
ρ is the density (kg/m³),
m is the mass (kg),
V is the volume (m³).
Key Points:
Density is a scalar quantity.
Commonly used to characterize fluids and compare them to each other.
Pressure
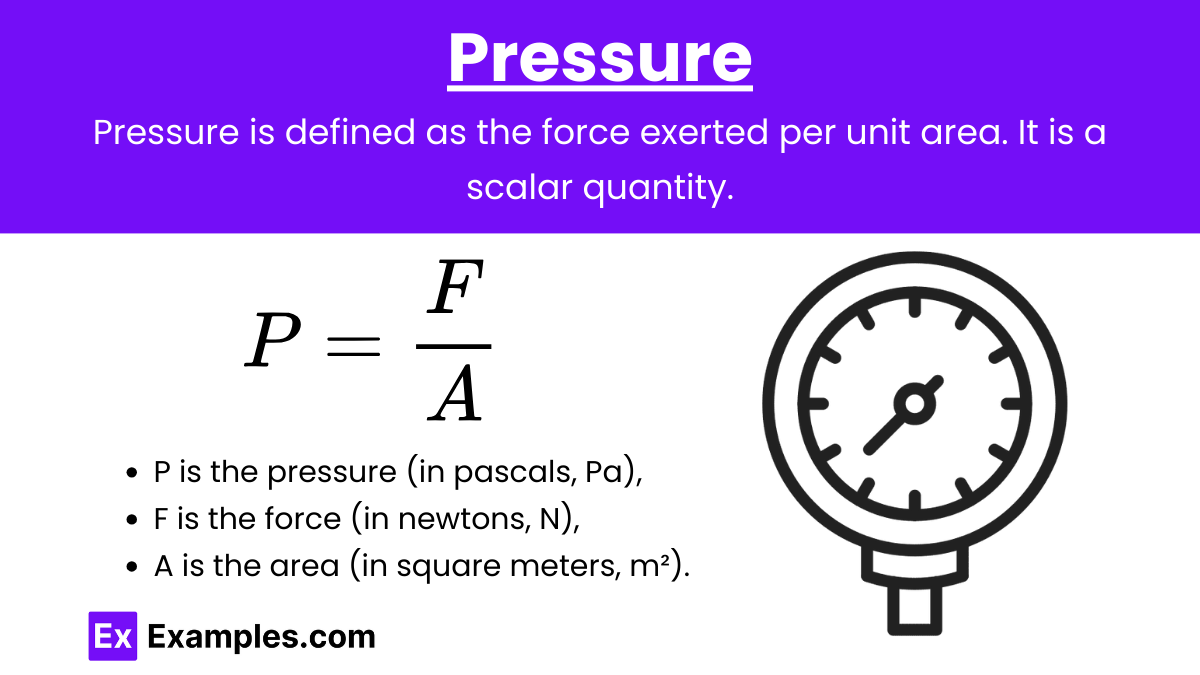
Pressure (P): Pressure is defined as the force exerted per unit area.
Formula: P = \frac{F}{A}
where:
P is the pressure (Pa or N/m²),
F is the force (N),
A is the area (m²).
Key Points:
Pressure in a fluid at rest is isotropic (same in all directions).
Increases with depth in a fluid due to the weight of the fluid above.
Pascal's Principle
Pascal's Principle: A change in pressure applied to an enclosed fluid is transmitted undiminished to all portions of the fluid and to the walls of its container.
Key Points:
Basis for hydraulic systems.
Applied in devices like hydraulic presses and car brakes.
Hydrostatic Pressure
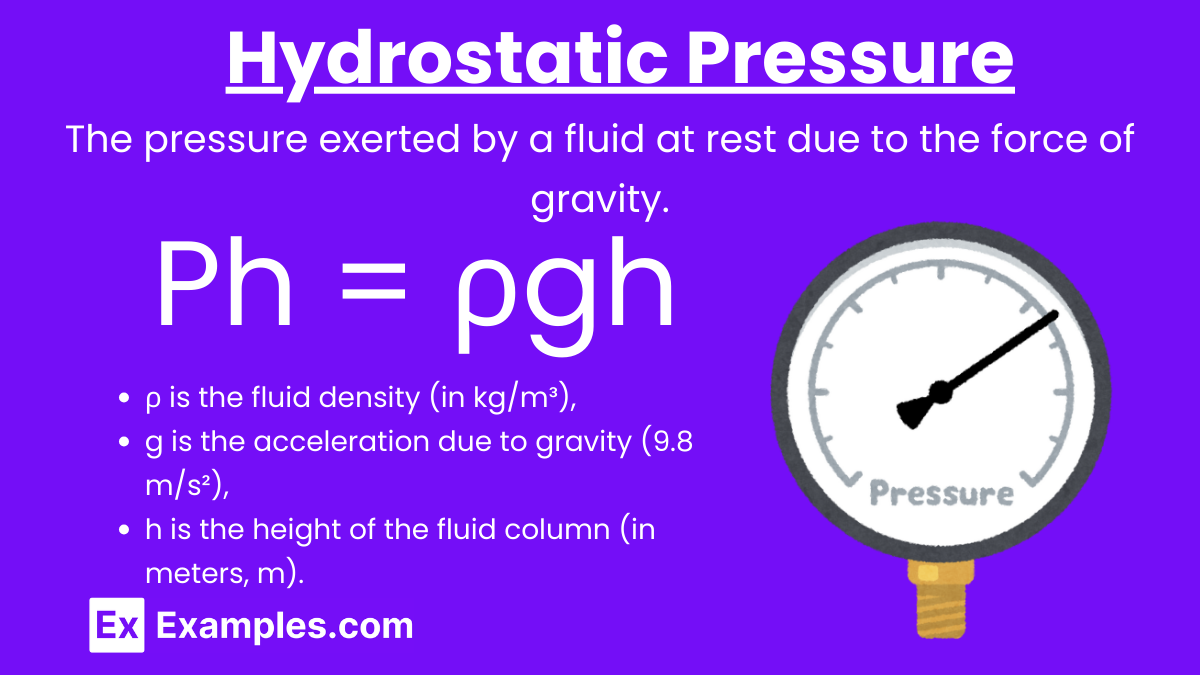
Hydrostatic Pressure (Pₕ): The pressure exerted by a fluid at rest due to the force of gravity.
Formula: Ph = ρgh
where:
ρ is the fluid density (in kg/m³),
g is the acceleration due to gravity (9.8 m/s²),
h is the height of the fluid column (in meters, m).
Key Points:
Increases linearly with depth.
Independent of the shape of the container.
Archimedes' Principle
Archimedes' Principle: An object submerged in a fluid experiences a buoyant force equal to the weight of the fluid displaced by the object.
Buoyant Force (Fb): F_b = \rho_{\text{fluid}} V_{\text{displaced}} g
where:
ρfluid is the fluid density (kg/m³),
Vdisplaced is the volume of fluid displaced (m³),
g is the acceleration due to gravity (9.8 m/s²).
Key Points:
Explains why objects float or sink.
Used in designing ships and submarines.
Fluid Dynamics
Continuity Equation
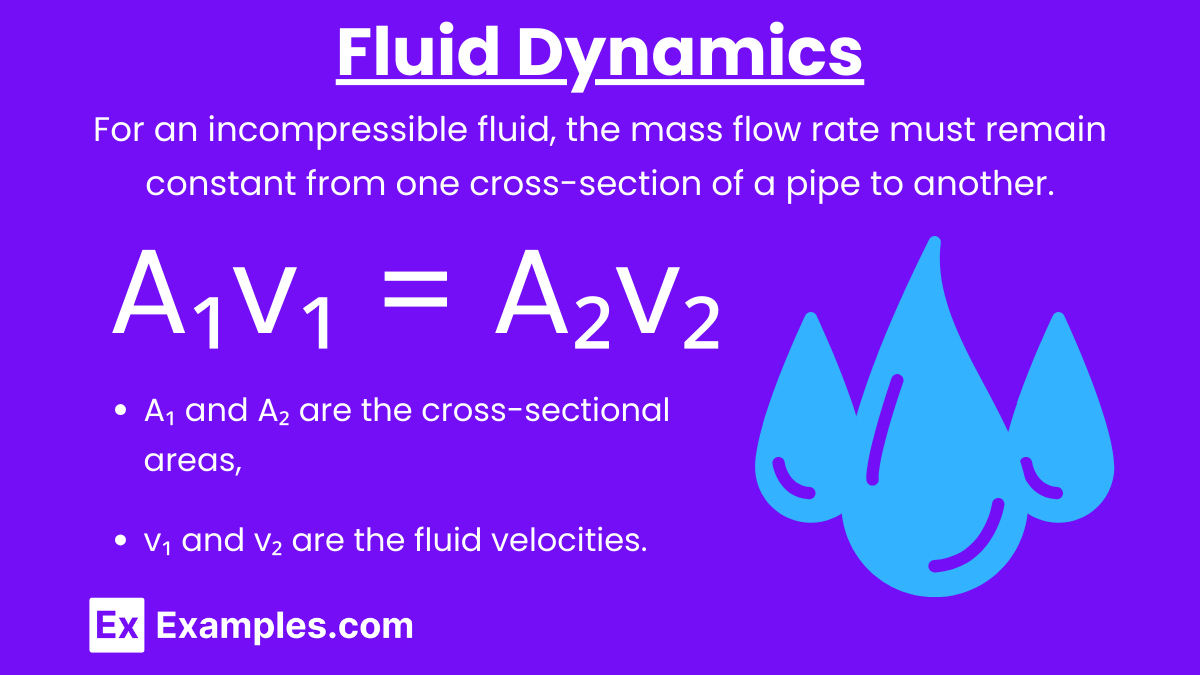
Continuity Equation: The mass flow rate of a fluid must remain constant from one cross-section of a pipe to another, assuming incompressible flow.
Formula: A₁v₁ = A₂v₂
where:
A₁ and A₂ are the cross-sectional areas (m²),
v₁ and v₂ are the flow velocities (m/s).
Key Points:
Ensures mass conservation in fluid flow.
Used to analyze varying pipe diameters.
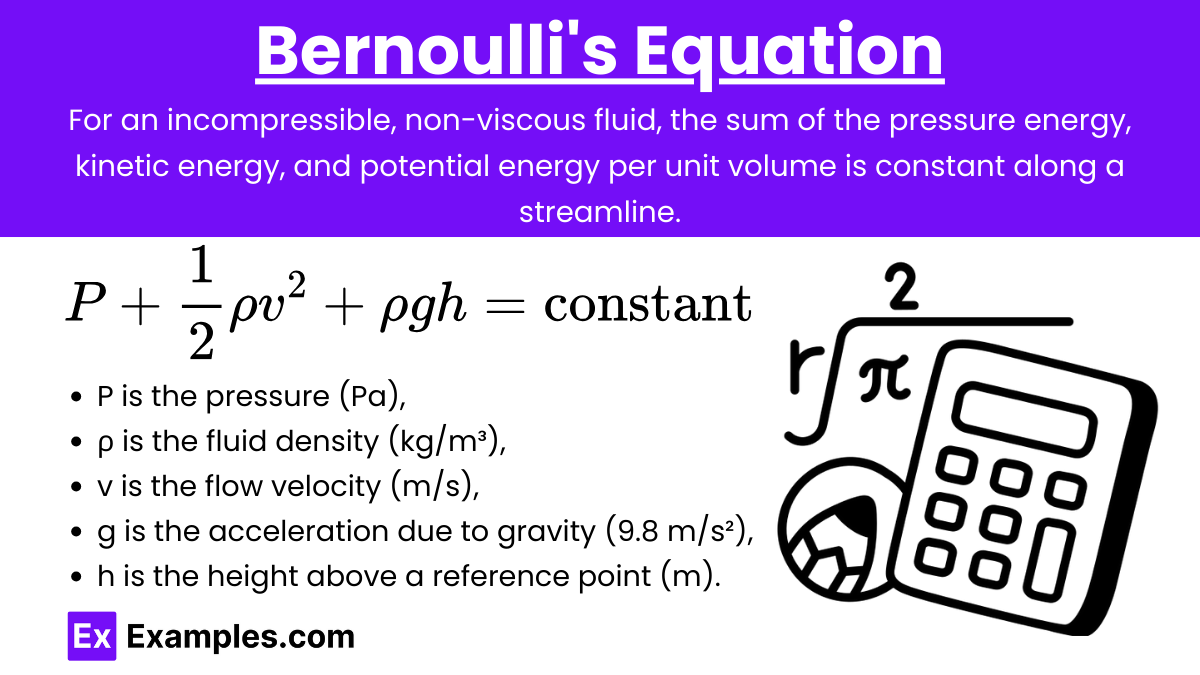
Bernoulli's Equation
Bernoulli's Equation: For an incompressible, non-viscous fluid, the sum of the pressure energy, kinetic energy, and potential energy per unit volume is constant along a streamline.
Formula: P + \frac{1}{2} \rho v^2 + \rho gh = \text{constant}
where:
P is the pressure (Pa),
ρ is the fluid density (kg/m³),
v is the flow velocity (m/s),
g is the acceleration due to gravity (9.8 m/s²),
h is the height above a reference point (m).
Key Points:
Describes the conservation of energy in fluid flow.
Explains phenomena like the Venturi effect and lift in airplane wings.
Examples
Example 1: Calculating Pressure at a Depth
Scenario: Calculate the pressure at a depth of 10 meters in a lake, assuming the atmospheric pressure at the surface is 1.01×10⁵ Pa and the density of water is 1000 kg/m³.
Solution: P = P_0 + \rho gh P = 1.01 \times 10^5 \, \text{Pa} + (1000 \, \text{kg/m}^3)(9.8 \, \text{m/s}^2)(10 \, \text{m}) P = 1.01 \times 10^5 \, \text{Pa} + 9.8 \times 10^4 \, \text{Pa} P = 2.01 \times 10^5 \, \text{Pa}
Example 2: Buoyant Force on a Submerged Object
Scenario: A 5 kg object is fully submerged in water. Calculate the buoyant force acting on the object.
Solution: F_b = \rho_{\text{fluid}} V_{\text{displaced}} g
Since the object is fully submerged, its volume is the same as the volume of water displaced. Let’s assume the volume of the object is 0.005 m³.
F_b = (1000 \, \text{kg/m}^3)(0.005 \, \text{m}^3)(9.8 \, \text{m/s}^2) F_b = 49 \, \text{N}
Example 3: Flow Speed in a Constricted Pipe
Scenario: Water flows through a pipe with a diameter of 10 cm at a speed of 2 m/s. If the pipe narrows to a diameter of 5 cm, what is the flow speed in the narrower section?
Solution: A_1 v_1 = A_2 v_2 \pi \left( \frac{0.1 \, \text{m}}{2} \right)^2 (2 \, \text{m/s}) = \pi \left( \frac{0.05 \, \text{m}}{2} \right)^2 v_2 \frac{\pi \left( 0.05 \, \text{m} \right)^2}{\pi \left( 0.025 \, \text{m} \right)^2} (2 \, \text{m/s}) = v_2 4 \times (2 \, \text{m/s}) = v_2 v_2 = 8 \, \text{m/s}
Example 4: Pressure in a Liquid
Problem:
A tank is filled with water to a height of 10 meters. What is the pressure at the bottom of the tank? (Assume the density of water is 1000 kg/m³ and gravitational acceleration is 9.8 m/s².)
Solution:
P = ρgh
P = 1000kg/m³×9.8m/s²×10m
P = 98,000Pa
Example 5: Continuity Equation
Problem:
Water flows through a pipe with a diameter of 10 cm at a speed of 2 m/s. The pipe then narrows to a diameter of 5 cm. What is the speed of the water in the narrower section of the pipe?
Solution:
A_1 v_1 = A_2 v_2 \pi (0.05 \, \text{m})^2 \times 2 \, \text{m/s} = \pi (0.025 \, \text{m})^2 \times v_2 v_2 = \frac{\pi (0.05)^2 \times 2}{\pi (0.025)^2} v_2 = \frac{0.0025 \times 2}{0.000625} v_2 = 8 \, \text{m/s}
Practice Problems
Question 1:
A pipe with a diameter of 0.2 meters carries water at a speed of 2 m/s. What is the flow rate of the water through the pipe?
A) 0.063 m³/s
B) 0.078 m³/s
C) 0.1 m³/s
D) 0.126 m³/s
Answer: A) 0.063 m³/s
Explanation:
The flow rate Q can be found using the formula:
Q = A×v
where A is the cross-sectional area of the pipe and v is the velocity of the fluid.
First, calculate the cross-sectional area A of the pipe:
A = \pi \left( \frac{d}{2} \right)^2 A = \pi \left( \frac{0.2 \, \text{m}}{2} \right)^2 A = \pi \times (0.1 \, \text{m})^2 A = \pi \times 0.01 \, \text{m}^2 A = 0.0314 \, \text{m}^2
Now, calculate the flow rate Q:
Q = 0.0314m²×2m/s = 0.0628m³/s≈0.063m³/s
Thus, the correct answer is 0.063 m³/s.
Question 2:
A tank contains 500 liters of oil with a density of 800 kg/m³. What is the pressure at the bottom of the tank due to the oil, assuming the height of the oil column is 1 meter?
A) 0.8 kPa
B) 4.9 kPa
C) 7.8 kPa
D) 9.8 kPa
Answer: B) 7.8 kPa
Explanation:
The pressure at the bottom of the tank due to the oil can be found using the hydrostatic pressure formula:
P = ρgh
where ρ is the density of the oil, g is the acceleration due to gravity (approximately 9.8m/s2), and h is the height of the oil column.
Given:
ρ = 800kg/m³
g = 9.8m/s²
h = 1m
Calculate the pressure:
P=800kg/m³×9.8m/s²×1m
P=7840Pa
P=7.84kPa≈7.8kPa
Thus, the correct answer is 7.8 kPa.
Question 3:
A fluid flows through a horizontal pipe with a varying cross-sectional area. At a point where the cross-sectional area is 0.05 m², the fluid velocity is 4 m/s. What is the velocity of the fluid at another point where the cross-sectional area is 0.02 m²?
A) 2 m/s
B) 6 m/s
C) 10 m/s
D) 20 m/s
Answer: D) 10 m/s
Explanation:
The continuity equation for fluid flow states that the flow rate must remain constant throughout the pipe:
A₁v₁ = A₂v₂
where A₁ and A₂ are the cross-sectional areas at points 1 and 2, and v₁ and v₂ are the fluid velocities at points 1 and 2, respectively.
Given:
A₁ = 0.05m²
v₁ = 4m/s
A₂ = 0.02m²
Solve for v₂:
0.05 \, \text{m}^2 \times 4 \, \text{m/s} = 0.02 \, \text{m}^2 \times v_2 0.2 \, \text{m}^3/\text{s} = 0.02 \, \text{m}^2 \times v_2 v_2 = \frac{0.2 \, \text{m}^3/\text{s}}{0.02 \, \text{m}^2} v_2 = 10 \, \text{m/s}
Thus, the correct answer is 10 m/s.