Resistivity and Resistance

Last Updated: September 24, 2024

Notes

Understanding resistivity and resistance is crucial for mastering the concepts of electricity and circuits in the AP Physics exam. These concepts explain how materials resist the flow of electric current and are fundamental to analyzing electrical circuits. Below are detailed notes on resistivity and resistance along with five examples to help you achieve a high score on your AP Physics exam.

Free AP Physics 2: Algebra-Based Practice Test

Learning Objectives

For the AP Physics exam, you should learn the concepts of resistivity and resistance, understanding how they differ and how they are related. Master Ohm’s Law and its applications in calculating resistance, voltage, and current in electrical circuits. Explore how resistivity depends on material properties and temperature. Study the factors affecting resistance, such as length, cross-sectional area, and material type. Learn to interpret and analyze graphs related to resistivity and resistance, and apply these principles to solve circuit problems.

Resistivity

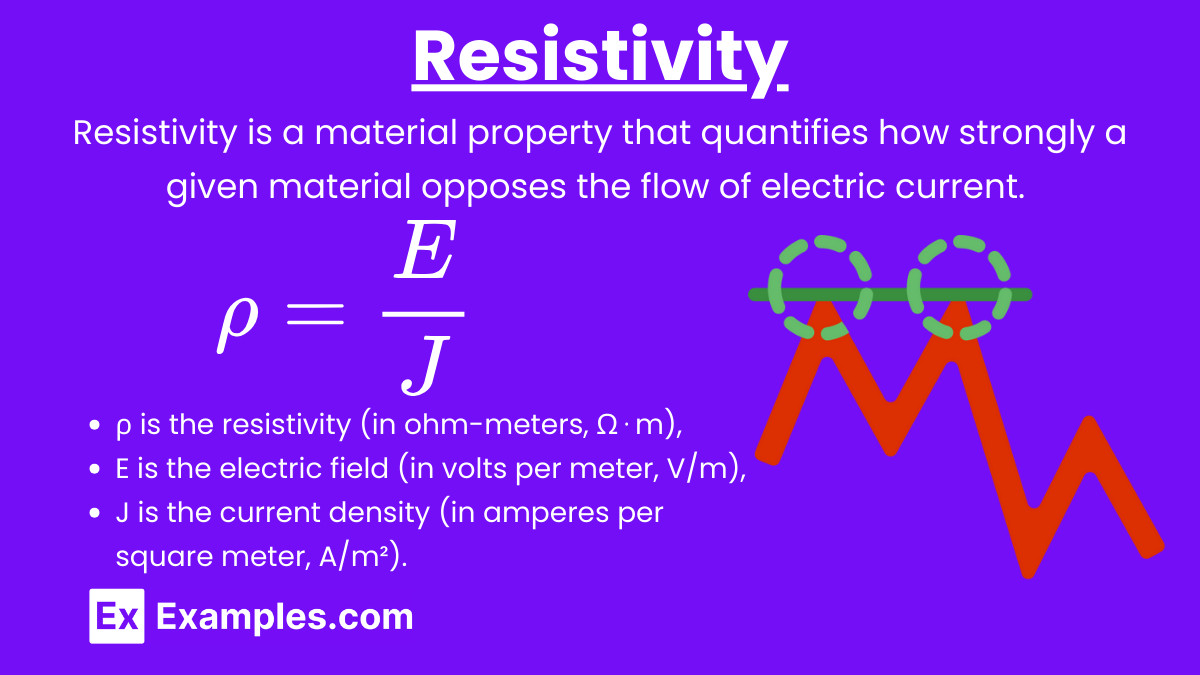
Resistivity (ρ): Resistivity is a material property that quantifies how strongly a given material opposes the flow of electric current. It is dependent on the material and its temperature.

Formula:

$\rho = \frac{E}{J}$

where:

ρ is the resistivity (in ohm-meters, Ω⋅m),
E is the electric field (in volts per meter, V/m),
J is the current density (in amperes per square meter, A/m²).

Key Points:

Materials with low resistivity are good conductors (e.g., metals).
Materials with high resistivity are good insulators (e.g., rubber, glass).

Resistance

Resistance (R): Resistance is a measure of the opposition to the flow of electric current in an electrical component. It is dependent on the material’s resistivity, the length of the conductor, and its cross-sectional area.

Ohm’s Law: V = IR

where:

V is the voltage (in volts, V),
I is the current (in amperes, A),
R is the resistance (in ohms, Ω ).

Formula for Resistance:

$R = \rho \frac{L}{A}$

where:

R is the resistance,
ρ is the resistivity,
L is the length of the conductor (in meters, m),
A is the cross-sectional area of the conductor (in square meters, m²).

Key Points:

Resistance increases with length and decreases with cross-sectional area.
Resistance is also affected by temperature; for most materials, resistance increases with temperature.

Example 1

Calculating Resistance of a Wire

Scenario: A copper wire (resistivity 1.68×10⁻⁸Ω⋅m) is 2 meters long and has a cross-sectional area of 1×10⁻⁶m². Calculate the resistance of the wire.

Solution:

$R = \rho \frac{L}{A}$

$R = 1.68 \times 10^{-8} \frac{2}{1 \times 10^{-6}}$

$R = 1.68 \times 10^{-8} \times 2 \times 10^{6}$

$R = 3.36 \times 10^{-2} \, \Omega$

Example 2

Temperature Dependence of Resistance

Scenario: The resistance of a tungsten wire at 20°C is 5 ohms. The temperature coefficient of resistivity for tungsten is 4.5×10⁻³°C⁻¹. Calculate the resistance of the wire at 100°C.

Solution:

$R_T = R_0 [1 + \alpha (T - T_0)]$

$R_{100} = 5[1 + 4.5 \times 10^{-3} (100 - 20)]$

$R_{100} = 5[1 + 4.5 \times 10^{-3} \times 80]$

$R_{100} = 5[1 + 0.36]$

$R_{100} = 5 \times 1.36$

$R_{100} = 6.8 \, \Omega$

Example 3

Comparing Resistances of Different Materials

Scenario: A 1 meter long aluminum wire (resistivity 2.82×10⁻⁸Ω⋅m) and a 1 meter long silver wire (resistivity 1.59×10⁻⁸Ω⋅m) both have a cross-sectional area of 2×10⁻⁶m². Calculate their resistances.

Solution for Aluminum:

$R_{\text{Al}} = \rho \frac{L}{A}$

$R_{\text{Al}} = 2.82 \times 10^{-8} \frac{1}{2 \times 10^{-6}}$

$R_{\text{Al}} = 2.82 \times 10^{-8} \times 5 \times 10^{5}$

$R_{\text{Al}} = 1.41 \times 10^{-2} \, \Omega$

Solution for Silver:

$R_{\text{Ag}} = \rho \frac{L}{A}$

$R_{\text{Ag}} = 1.59 \times 10^{-8} \frac{1}{2 \times 10^{-6}}$

$R_{\text{Ag}} = 1.59 \times 10^{-8} \times 5 \times 10^{5}$

$R_{\text{Ag}} = 7.95 \times 10^{-3} \, \Omega$

Example 4

Determining Length for a Specific Resistance

Scenario: You have a wire made of nickel (resistivity 6.99×10⁻⁸Ω⋅m) with a cross-sectional area of 3×10⁻⁶m². You need the wire to have a resistance of 0.5Ω. Calculate the required length of the wire.

Solution:

$R = \rho \frac{L}{A}$

$L = \frac{RA}{\rho}$

$L = \frac{0.5 \times 3 \times 10^{-6}}{6.99 \times 10^{-8}}$

$L = \frac{1.5 \times 10^{-6}}{6.99 \times 10^{-8}}$

$L \approx 21.46 \, \text{m}$

Example 5

Power Dissipated in a Resistor

Scenario: A 10 ohm resistor is connected across a 12V battery. Calculate the power dissipated by the resistor.

Solution:

$P = \frac{V^2}{R}$

$P = \frac{12^2}{10}$

$P = \frac{144}{10}$

$P = 14.4 \, \text{W}$

Practice Problems

Question 1:

A copper wire has a length of 2 meters and a cross-sectional area of 1mm². If the resistivity of copper is 1.68×10⁻⁸Ω⋅m, what is the resistance of the wire?

A) 0.034Ω
B) 0.0168Ω
C) 0.00336Ω
D) 0.168Ω

Answer: C) 0.00336Ω

Explanation:

The resistance R of a wire is calculated using the formula:

$R = \rho \frac{L}{A}$

where ρ is the resistivity, L is the length, and A is the cross-sectional area.

Given:

ρ = 1.68×10⁻⁸Ω⋅m
L = 2m
A = 1mm² = 1×10⁻⁶m²

Calculate the resistance:

$R = 1.68 \times 10^{-8} \frac{2}{1 \times 10^{-6}} = 1.68 \times 10^{-8} \times 2 \times 10^{6} = 3.36 \times 10^{-3} \Omega = 0.00336 \Omega$

Thus, the correct answer is 0.00336Ω.

Question 2:

Which of the following factors does NOT affect the resistivity of a material?

A) Temperature
B) Length
C) Type of material
D) None of the above

Answer: B) Length

Explanation:

Resistivity is an intrinsic property of a material that depends on the type of material and its temperature but does not depend on the length or cross-sectional area of the material. The resistance of a material, however, depends on both the length and the cross-sectional area. Therefore, length does not affect the resistivity of a material.

Question 3:

A wire of material A has a resistivity twice that of material B. If both wires have the same length and cross-sectional area, how does the resistance of wire A compare to wire B?

A) The resistance of wire A is half that of wire B.
B) The resistance of wire A is the same as wire B.
C) The resistance of wire A is twice that of wire B.
D) The resistance of wire A is four times that of wire B.

Answer: C) The resistance of wire A is twice that of wire B.

Explanation:

The resistance R of a wire is given by:

$R = \rho \frac{L}{A}$

where ρ is the resistivity, L is the length, and A is the cross-sectional area. If the resistivity of wire A (ρₐ) is twice that of wire B (ρB) and both wires have the same length and cross-sectional area, the resistance of wire A will be:

$R_A = 2 \rho_B \frac{L}{A} = 2 \times R_B$

Thus, the resistance of wire A is twice that of wire B.