Radioactive Decay

Read radioactive decay is crucial. This process involves unstable atomic nuclei losing energy by emitting radiation, such as alpha particles, beta particles, or gamma rays. Radioactive decay is random and spontaneous, governed by principles like half-life and decay constants. Mastery of this topic includes calculating decay rates, solving problems on remaining nuclei and activity, and exploring applications in fields like carbon dating, medicine, and nuclear power. Proficiency in radioactive decay is essential for excelling in AP Physics.

Learning Objectives

Learn the types of radioactive decay (alpha, beta, gamma), how to calculate decay rates using half-life and decay constants, and the mathematical representation of decay processes. Learn to solve problems involving remaining nuclei, activity, and applications such as carbon dating and medical uses. Grasp safety measures for handling radioactive materials and comprehend real-world applications in energy production and radiation safety. Practice solving related problems to ensure a strong conceptual and practical understanding.

Radioactive Decay

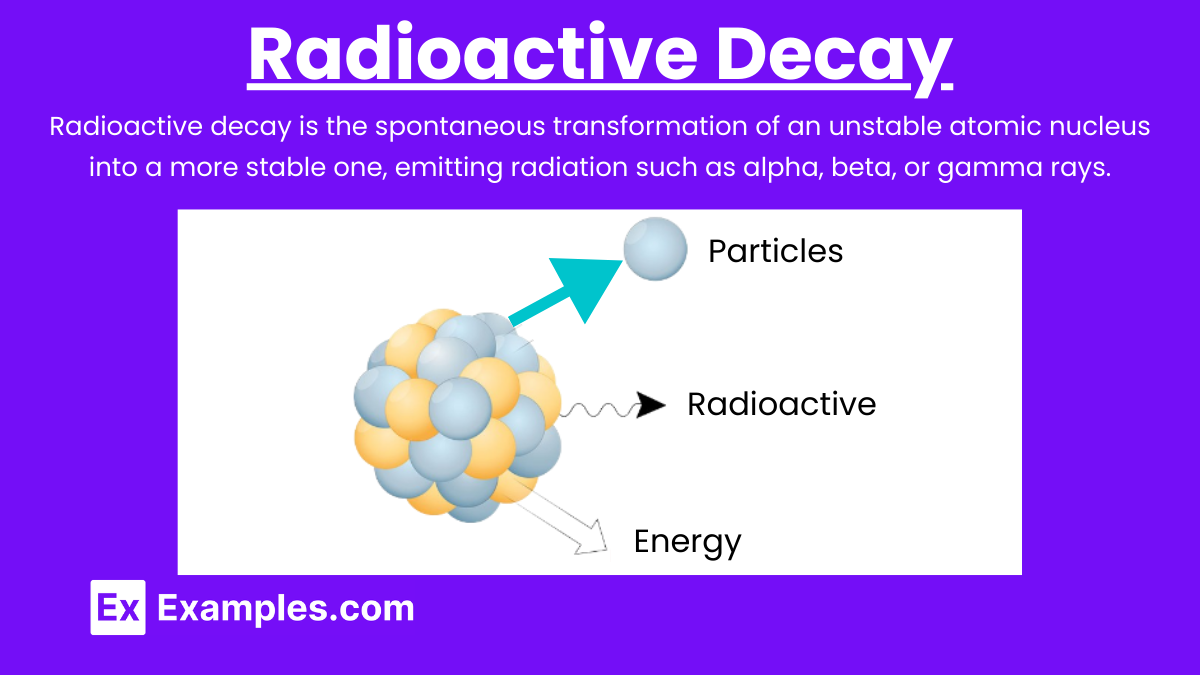
Radioactive decay is the process by which an unstable atomic nucleus loses energy by emitting radiation. This radiation can be in the form of alpha particles, beta particles, or gamma rays. The process is random and spontaneous, meaning it is impossible to predict when a particular nucleus will decay.

Types of Radioactive Decay

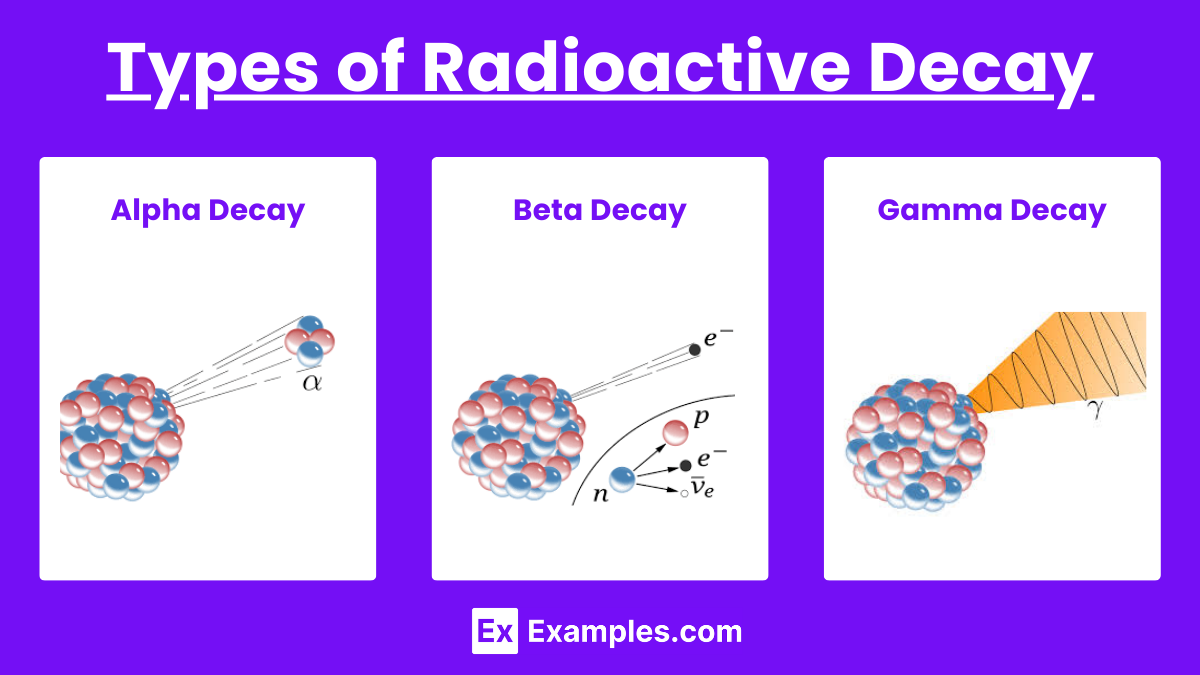
1. Alpha Decay
   • An alpha particle (2 protons and 2 neutrons) is emitted from the nucleus.
   • Decreases the atomic number by 2 and the mass number by 4.
   • Example: Uranium-238 decays to Thorium-234.
2. Beta Decay
   • A beta particle (electron or positron) is emitted.
   • Increases or decreases the atomic number by 1 without changing the mass number.
   • Types:
     • Beta-minus (β-) decay: A neutron is converted to a proton, and an electron is emitted.
       • Example: Carbon-14 decays to Nitrogen-14.
     • Beta-plus (β+) decay (Positron emission): A proton is converted to a neutron, and a positron is emitted.
       • Example: Sodium-22 decays to Neon-22.
3. Gamma Decay
   • Emission of gamma rays (high-energy photons).
   • Usually follows alpha or beta decay to release excess energy.
   • Does not change the atomic or mass numbers.
   • Example: Cobalt-60 emits gamma rays after beta decay.

Key Concepts

Half-Life

• Half-life (T₁/₂) is the time required for half of the radioactive nuclei in a sample to decay.
• Each isotope has a unique half-life, ranging from fractions of a second to billions of years.
• Example: The half-life of Carbon-14 is approximately 5,730 years.

Decay Constant

• The decay constant (λ) represents the probability of decay of a nucleus per unit time.
• It is related to the half-life by the equation: \[T_{1/2} = \frac{\ln(2)}{\lambda}\]

Activity

• Activity (A) is the number of decays per unit time in a radioactive sample.
• Measured in becquerels (Bq) or curies (Ci).
• Calculated by the equation: A=λN where N is the number of undecayed nuclei.

Mathematical Representation

Radioactive decay follows first-order kinetics and can be described by the following equations:

1. Number of Nuclei Remaining (N) \[N(t) = N_0 e^{-\lambda t}\]
   • N(t) is the number of nuclei at time ttt.
   • N0​ is the initial number of nuclei.
   • λ is the decay constant.
   • t is the time elapsed.
2. Activity (A) \[A(t)=A0e−λt\]
   • A(t)A(t)A(t) is the activity at time ttt.
   • A0A_0A0​ is the initial activity.

Examples of Radioactive Decay

1. Uranium-238 to Thorium-234 (Alpha Decay)
   • Uranium-238 emits an alpha particle, transforming into Thorium-234.
2. Carbon-14 to Nitrogen-14 (Beta-Minus Decay)
   • Carbon-14 decays by emitting a beta particle (electron), becoming Nitrogen-14.
3. Sodium-22 to Neon-22 (Beta-Plus Decay)
   • Sodium-22 emits a positron, resulting in Neon-22.
4. Cobalt-60 Emission of Gamma Rays
   • After beta decay, Cobalt-60 releases excess energy as gamma rays.
5. Radon-222 to Polonium-218 (Alpha Decay)
   • Radon-222 undergoes alpha decay to form Polonium-218.

Practice Test Questions on Radioactive Decay

Question 1

Which of the following remains conserved during radioactive decay?

A) Mass-Energy

B) Number of protons

C) Number of neutrons

D) All of the above

Answer: A) Mass-Energy

Explanation: In radioactive decay, the total mass-energy is conserved. While the number of protons and neutrons can change (e.g., a neutron converting to a proton in beta-minus decay), the overall energy, including the rest mass of particles and emitted radiation, remains constant. This principle aligns with the law of conservation of mass-energy, which states that the total amount of mass and energy in a closed system remains constant over time.

Question 2

During alpha decay, which of the following is conserved?

A) Atomic number

B) Total charge

C) Number of neutrons

D) None of the above

Answer: B) Total charge

Explanation: In alpha decay, an alpha particle (comprising 2 protons and 2 neutrons) is emitted from the nucleus. The atomic number of the original nucleus decreases by 2, and the mass number decreases by 4. However, the total charge is conserved because the loss of two protons from the parent nucleus is balanced by the charge of the emitted alpha particle (+2).

Question 3

In beta-minus decay, what happens to the neutron-to-proton ratio in the nucleus?

A) Increases

B) Decreases

C) Remains the same

D) Becomes zero

Answer: B) Decreases

Explanation: In beta-minus decay, a neutron in the nucleus converts into a proton and an electron. The electron (beta particle) is emitted, and the proton remains in the nucleus. This process increases the number of protons while decreasing the number of neutrons, thereby decreasing the neutron-to-proton ratio in the nucleus. This change helps stabilize the nucleus by moving it closer to the ideal neutron-to-proton ratio for a stable isotope.