Kirchhoff’s Junction Rule and the Conservation of Electric Charge

Last Updated: September 24, 2024

Notes

Understanding Kirchhoff’s Junction Rule and the conservation of electric charge is essential for mastering electrical circuits in the AP Physics exam. These principles are fundamental in analyzing complex circuits involving multiple branches and junctions. Below are detailed notes on Kirchhoff’s Junction Rule and the conservation of electric charge, along with five examples to help you achieve a high score on your AP Physics exam.

Free AP Physics 2: Algebra-Based Practice Test

Learning Objectives

By studying Kirchhoff’s Junction Rule and the Conservation of Electric Charge for the AP Physics exam, you should be able to understand and apply the principle that the total current entering a junction equals the total current leaving the junction. You should also grasp how this rule is a direct consequence of the conservation of electric charge. Mastery of this topic includes solving complex circuit problems, analyzing current flow in multiple branches, and recognizing the importance of charge conservation in electrical circuits.

Kirchhoff’s Junction Rule

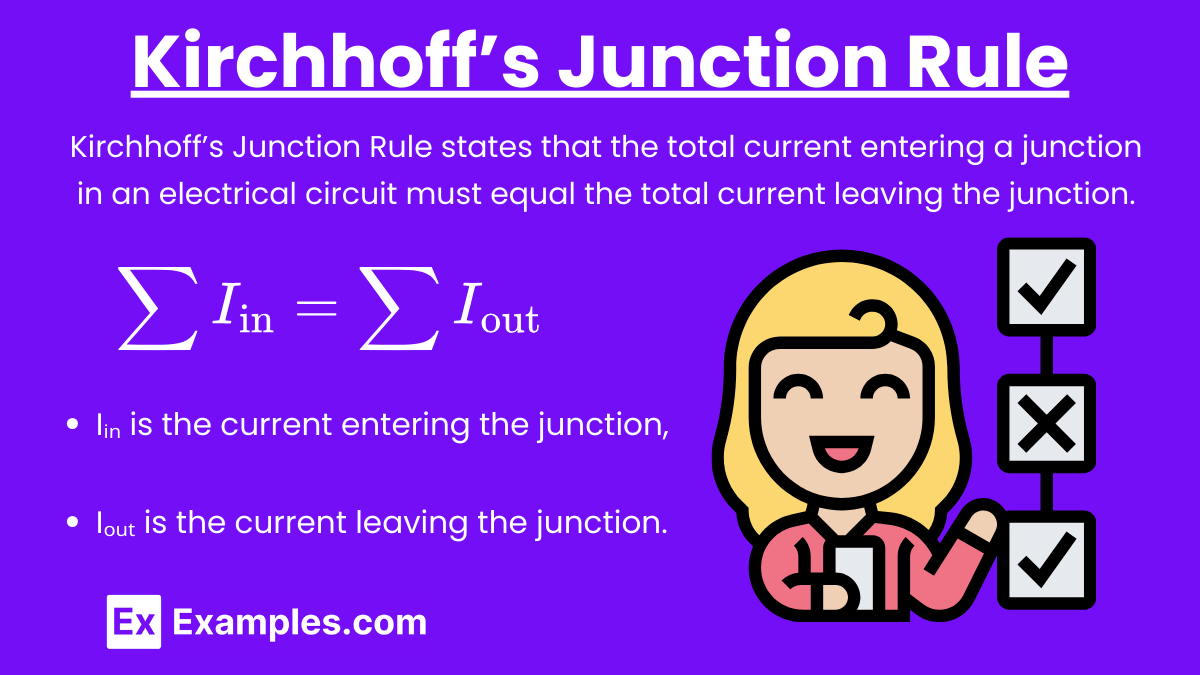
Kirchhoff’s Junction Rule (Current Law): Kirchhoff’s Junction Rule states that the total current entering a junction (or node) in an electrical circuit must equal the total current leaving the junction. This rule is based on the conservation of electric charge.

Mathematical Formulation: $\sum I_{\text{in}} = \sum I_{\text{out}}$

where:

Iᵢₙ is the current entering the junction,
Iₒᵤₜ is the current leaving the junction.

Key Points:

The sum of currents entering a junction equals the sum of currents leaving the junction.
This rule is derived from the principle of conservation of electric charge.

Conservation of Electric Charge

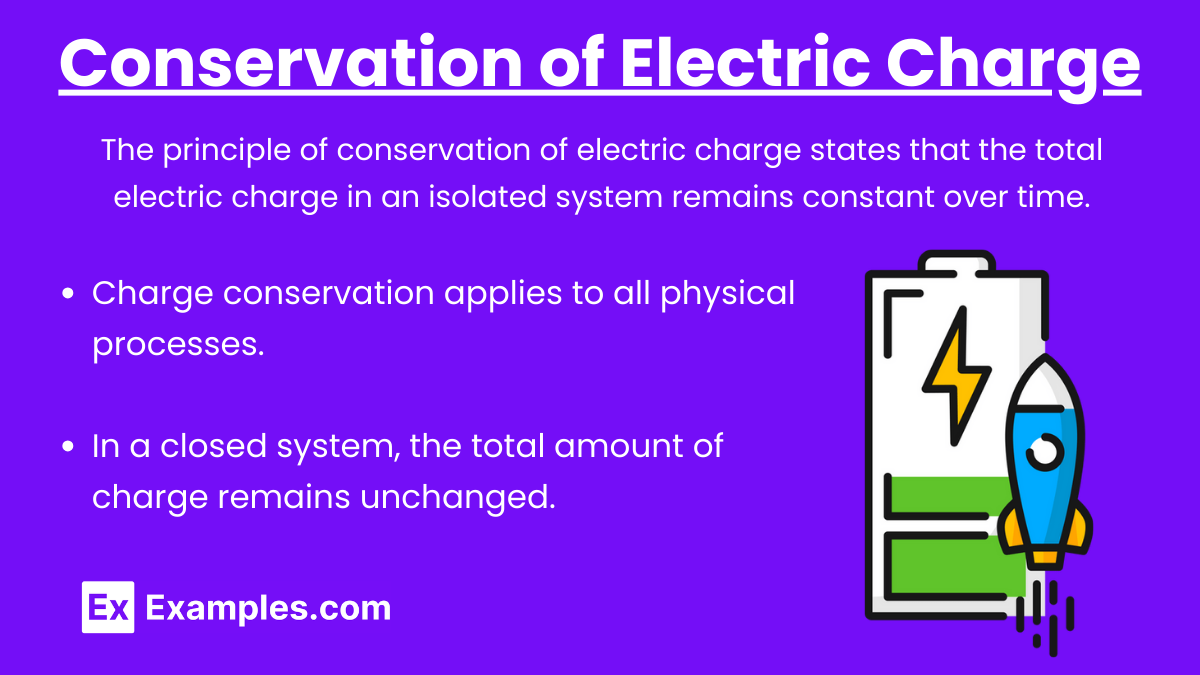
Conservation of Electric Charge: The principle of conservation of electric charge states that the total electric charge in an isolated system remains constant over time. Electric charge can neither be created nor destroyed; it can only be transferred from one part of the system to another.

Key Points:

Charge conservation applies to all physical processes.
In a closed system, the total amount of charge remains unchanged.

Example 1

Simple Junction

Scenario: In a circuit, three wires meet at a junction. The currents in two of the wires are I₁ = 2A and I₂ = 3A, both flowing into the junction. Calculate the current I₃ flowing out of the junction.

Solution: Using Kirchhoff’s Junction Rule: $\sum I_{\text{in}} = \sum I_{\text{out}}$
$I_{1} + I_{2} = I_{3}$
$2 \, \text{A} + 3 \, \text{A} = I_{3}$
$I_{3} = 5 \, \text{A}$

Example 2

Complex Junction

Scenario: Four currents meet at a junction: I₁ = 1A entering, I₂ = 4A entering, I3 = 2A leaving, and I4 leaving. Calculate the current I4.

Solution: Using Kirchhoff’s Junction Rule: $\sum I_{\text{in}} = \sum I_{\text{out}}$
$I_{1} + I_{2} = I_{3} + I_{4}$
$1 \, \text{A} + 4 \, \text{A} = 2 \, \text{A} + I_{4}$
$5 \, \text{A} = 2 \, \text{A} + I_{4}$
$I_{4} = 3 \, \text{A}$

Example 3

Series and Parallel Circuits

Scenario: In a parallel circuit, a 12 V battery is connected to two resistors R₁ = 6Ω and R₂ = 3Ω. Calculate the total current supplied by the battery and the current through each resistor.

Solution:

Total Resistance in Parallel: $\frac{1}{R_{\text{total}}} = \frac{1}{R_{1}} + \frac{1}{R_{2}}$
$\frac{1}{R_{\text{total}}} = \frac{1}{6} + \frac{1}{3}$
$\frac{1}{R_{\text{total}}} = \frac{1}{6} + \frac{2}{6}$
$R_{\text{total}} = \frac{6}{3}$
$R_{\text{total}} = 2 \, \Omega$

Total Current: $I_{\text{total}} = \frac{V}{R_{\text{total}}}$
$I_{\text{total}} = \frac{12 \, V}{2 \, \Omega}$
$I_{\text{total}} = 6 \, A$

Current Through Each Resistor: Using Ohm’s Law (V=IR):
$I_{1} = \frac{V}{R_{1}} = \frac{12 \, V}{6 \, \Omega} = 2 \, A$
$I_{2} = \frac{V}{R_{2}} = \frac{12 \, V}{3 \, \Omega} = 4 \, A$

Verification Using Kirchhoff’s Junction Rule: $I_{\text{total}} = I_{1} + I_{2}$
$6 \, A = 2 \, A + 4 \, A$

Example 4

Circuit with Multiple Junctions

Scenario: In a circuit with multiple junctions, the currents at junction A are I₁ = 3A entering, I₂ = 2A entering, and I₃ leaving. At junction B, I₃ enters and splits into I₄ = 4A and I₅ = 1A. Find I₃ and verify Kirchhoff’s Junction Rule at both junctions.

Solution: At Junction A: $I_{1} + I_{2} = I_{3}$
$3 \, A + 2 \, A = I_{3}$
$I_{3} = 5 \, A$

At Junction B: $I_{3} = I_{4} + I_{5}$
$5 \, A = 4 \, A + 1 \, A$

Example 5

Applying Kirchhoff’s Rules to a Wheatstone Bridge

Scenario: In a Wheatstone bridge, resistors R₁ = 10Ω, R₂ = 10Ω, R₃ = 5Ω, and R₄ = 15Ω are connected. A battery of 12 V is connected across the bridge. Calculate the current through R₁ and R₂ if the bridge is balanced.

Solution: Condition for a Balanced Bridge: $\frac{R_{1}}{R_{2}} = \frac{R_{3}}{R_{4}}$
$\frac{10 \, \Omega}{10 \, \Omega} = \frac{5 \, \Omega}{15 \, \Omega}$
$1 \neq \frac{1}{3}$

Since the bridge is not balanced, we need to use Kirchhoff’s rules to solve the problem, but for simplicity, we can assume an approximation if the bridge were balanced.

For a balanced bridge, no current flows through the galvanometer, and the current through R1 and R2 can be calculated using Ohm’s Law and Kirchhoff’s rules.

Practice Problems

Question 1

In a circuit, three currents meet at a junction. If I₁ = 2A flows into the junction, I₂ = 3A flows out of the junction, what is the value of the third current I₃?

A) 1 A into the junction
B) 1 A out of the junction
C) 5 A into the junction
D) 5 A out of the junction

Answer: B) 1 A out of the junction

Explanation:

Kirchhoff’s Junction Rule states that the total current entering a junction must equal the total current leaving the junction. Mathematically, this can be expressed as:

Iᵢₙ = Iₒᵤₜ

Given:

I₁ = 2A (into the junction)
I₂ = 3A (out of the junction)

Let I₃ be the current we need to find. Applying Kirchhoff’s Junction Rule:

$I_{1} = I_{2} + I_{3}$
$2 \, A = 3 \, A + I_{3}$
$I_{3} = 2 \, A - 3 \, A$
$I_{3} = -1 \, A$

The negative sign indicates that I₃ flows out of the junction. Therefore, I₃ = 1A out of the junction.

Question 2

At a junction in a circuit, the currents flowing towards the junction are 5A and 7A, and the currents flowing away from the junction are 4A and 6A. What is the value of the missing current I?

A) 2 A towards the junction
B) 2 A away from the junction
C) 4 A towards the junction
D) 4 A away from the junction

Answer: A) 2 A towards the junction

Explanation:

Using Kirchhoff’s Junction Rule, we know that the total current entering the junction equals the total current leaving the junction:

Iᵢₙ = Iₒᵤₜ

Given:

Currents towards the junction: 5A, 7A
Currents away from the junction: 4A, 6A, and the unknown current I

Calculate the total currents: 5A+7A = 12A
4A+6A+I = 12A

So, solving for I:

12A=10A+I
I=12A−10A
I=2A

Therefore, the missing current I is 2 A towards the junction.

Question 3

In a complex circuit, a junction has four wires connected to it. Currents of 3A, 2A, and 1A flow into the junction. If the current flowing out of the junction is I, what is the value of I?

A) 2 A
B) 3 A
C) 6 A
D) 1 A

Answer: C) 6 A

Explanation:

Applying Kirchhoff’s Junction Rule, we need to ensure the total current entering the junction equals the total current leaving the junction.

Given:

Currents into the junction: 3A, 2A, 1A
Current out of the junction: I

Calculate the total currents: 3A+2A+1A = 6A

Therefore, the current I flowing out of the junction is:

I = 6A

Thus, the correct answer is 6 A.