Wave Function and Probability

Last Updated: September 24, 2024

Notes

The wave function is a core concept in quantum mechanics, describing the quantum state of a particle or system. It encapsulates all information about a system, allowing calculations of probabilities for various outcomes. For the AP Physics exam, mastering the wave function’s definition, properties, and probabilistic interpretation is crucial. Key aspects include the probability density ∣Ψ∣², wave function normalization, and the Schrödinger equation. Understanding these fundamentals is essential for excelling in AP Physics.

Free AP Physics 2: Algebra-Based Practice Test

Learning Objectives

Focus on understanding the definition and properties of the wave function, including its complex nature and normalization. Learn to interpret the probability density ∣Ψ∣² and calculate the probability of finding a particle in a specific region. Master the Schrödinger equation for wave function evolution and comprehend the concept of wave function collapse upon measurement. Additionally, grasp the Heisenberg uncertainty principle and be able to apply these concepts to various quantum systems.

Introduction to Wave Functions

In quantum mechanics, the wave function is a fundamental concept used to describe the quantum state of a particle or system of particles. It contains all the information about the system and can be used to calculate probabilities of finding particles in various positions or states.

Wave Function

A wave function (usually denoted by the Greek letter Ψ, psi) is a complex-valued function of space and time. For a single particle in one dimension, it is written as:

Ψ(x,t)

For three dimensions:

Ψ(r,t)

where r=(x,y,z) represents the position vector.

Properties of Wave Function

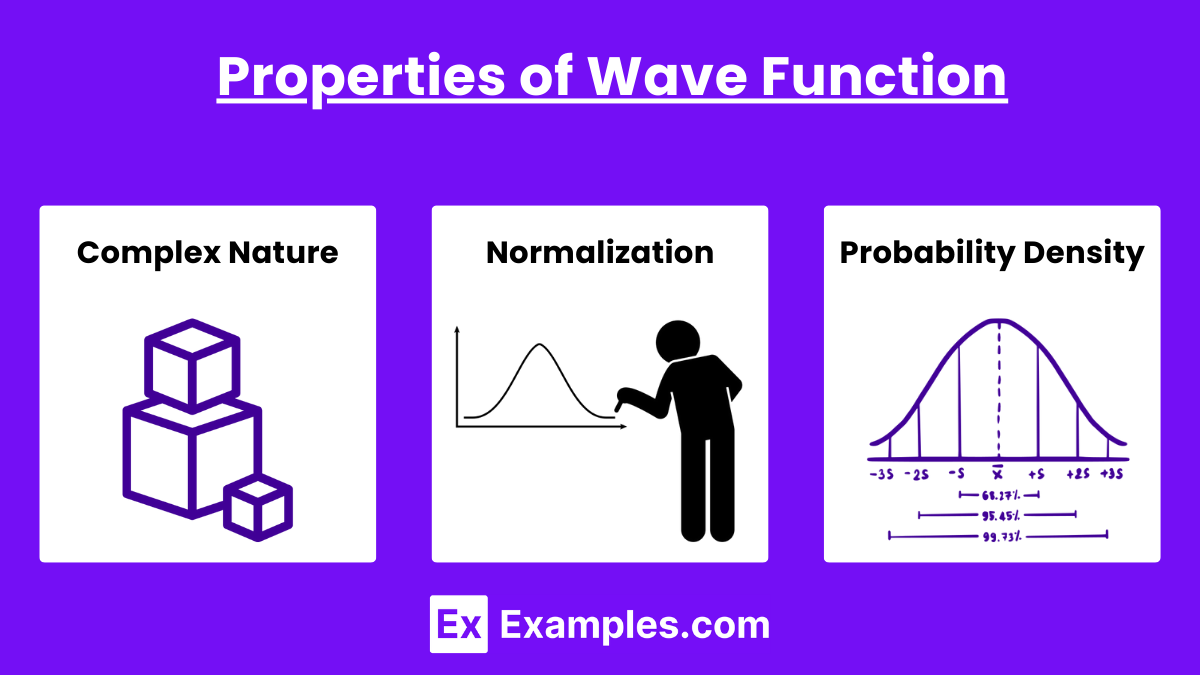
Complex Nature: The wave function is generally complex, meaning it has both real and imaginary components.
Normalization: The total probability of finding the particle somewhere in space must be 1, which leads to the normalization condition:

$\int_{-\infty}^{\infty} |\Psi(x, t)|^2 \, dx = 1$

Probability Density: The square of the absolute value of the wave function gives the probability density:

$P(x, t) = |\Psi(x, t)|^2$

This represents the probability of finding the particle at position xxx at time ttt.

Wave Function and Schrödinger Equation

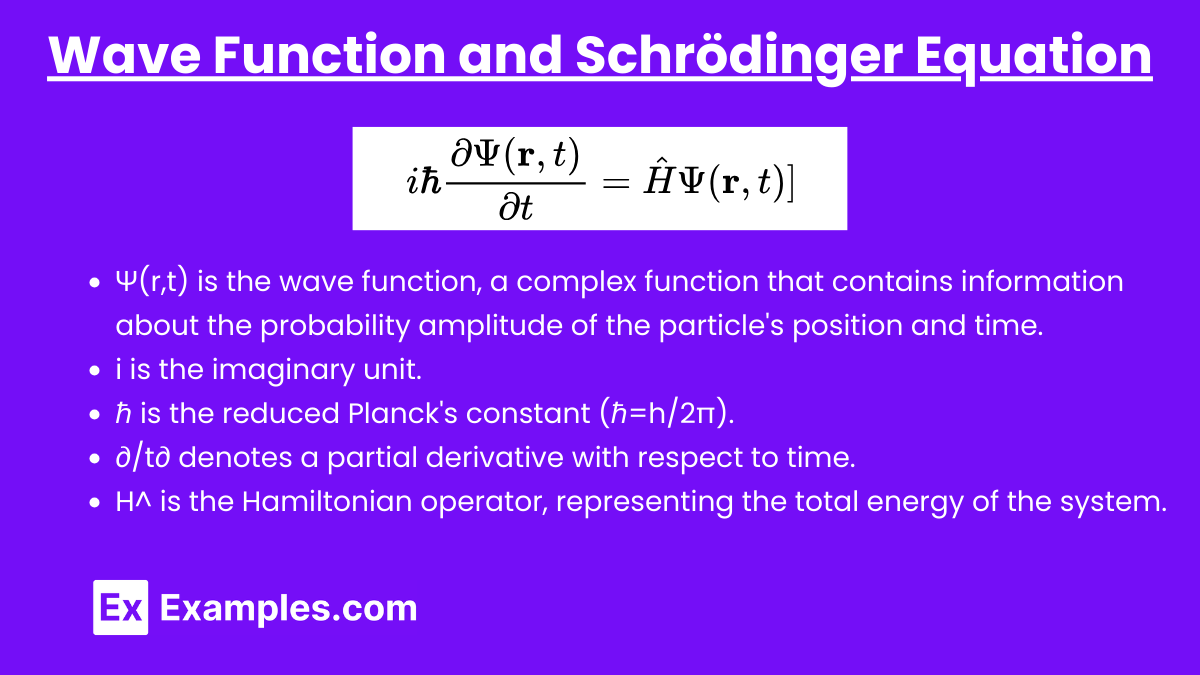
The wave function evolves over time according to the Schrödinger equation. For a non-relativistic particle, the time-dependent Schrödinger equation is:

$i \hbar \frac{\partial \Psi(\mathbf{r}, t)}{\partial t} = \hat{H} \Psi(\mathbf{r}, t)$

where ℏ\hbarℏ is the reduced Planck’s constant and H^\hat{H}H^ is the Hamiltonian operator, representing the total energy of the system.

Probability and Measurement in Quantum Mechanics

Probability Interpretation

In quantum mechanics, the outcome of a measurement is probabilistic. The wave function provides the probabilities of various outcomes:

Probability Density: As mentioned, ∣Ψ(x,t)∣² gives the probability density of finding a particle at position xxx at time ttt.
Expectation Values: The expectation value (average value) of an observable A^ can be calculated using the wave function:

$\langle \hat{A} \rangle = \int_{-\infty}^{\infty} \Psi^*(x, t) \hat{A} \Psi(x, t) \, dx$

where Ψ∗(x,t) is the complex conjugate of Ψ(x,t).

Probability of Finding a Particle in a Region

To find the probability of locating a particle within a specific region [a,b][a, b][a,b], integrate the probability density over that region:

$P(a \leq x \leq b, t) = \int_{a}^{b} |\Psi(x, t)|^2 \, dx$

Wave Function Collapse

Upon measurement, the wave function collapses to an eigenstate of the observable being measured. This collapse process changes the wave function instantaneously.

Key Concepts and Equations

Heisenberg Uncertainty Principle

One of the implications of wave functions and their probabilistic nature is the Heisenberg uncertainty principle. It states that it is impossible to simultaneously know both the position and momentum of a particle with infinite precision:

$\Delta x \Delta p \geq \frac{\hbar}{2}$

where Δx and Δp are the uncertainties in position and momentum, respectively.

Examples

Example 1: Particle in a Box

Consider a particle confined in a one-dimensional box of length LLL with infinite potential walls. The wave functions for the stationary states are:

$\Psi_n(x) = \sqrt{\frac{2}{L}} \sin \left( \frac{n \pi x}{L} \right)$

where n is a positive integer (quantum number).

The probability density is:

$|\Psi_n(x)|^2 = \frac{2}{L} \sin^2 \left( \frac{n \pi x}{L} \right)$

Example 2: Gaussian Wave Packet

A Gaussian wave packet is a localized wave function that describes a particle with a well-defined average position and momentum. Its wave function can be written as:

$\Psi(x, 0) = \left( \frac{1}{2 \pi \sigma^2} \right)^{1/4} \exp \left( -\frac{x^2}{4\sigma^2} \right)$

where σ is the standard deviation, determining the width of the packet.

Examples of Wave Function and Probability

Particle in a One-Dimensional Box:
- The wave function for a particle in a box of length L is Ψn(x)=
- $\sqrt{\frac{2}{L}} \sin \left( \frac{n \pi x}{L} \right)$
- The probability density is
  $|\Psi_n(x)|^2 = \frac{2}{L} \sin^2 \left( \frac{n \pi x}{L} \right)$
Harmonic Oscillator:
- For a quantum harmonic oscillator, the wave function for the ground state is
  $\Psi_0(x) = \left( \frac{m \omega}{\pi \hbar} \right)^{1/4} \exp \left( -\frac{m \omega x^2}{2 \hbar} \right)$
- The probability density
  $|\Psi_0(x)|^2$
  is a Gaussian distribution centered at x=0x = 0x=0.
Free Particle:
- A free particle’s wave function is given by a plane wave: Ψ(x,t)=Aei(kx−ωt)\Psi(x, t) = A e^{i(kx – \omega t)}Ψ(x,t)=
  $A e^{i(kx - \omega t)}$
- The probability density ∣Ψ(x,t)∣² is constant, indicating the particle is equally likely to be found anywhere.
Hydrogen Atom:
- The wave function for the electron in a hydrogen atom’s ground state is
  $\Psi_{100}(r) = \frac{1}{\sqrt{\pi a_0^3}} e^{-r/a_0}$
- where a0 is the Bohr radius.
- The probability density
  $|\Psi_{100}(r)|^2 = \frac{1}{\pi a_0^3} e^{-2r/a_0}$
  gives the likelihood of finding the electron at distance rrr from the nucleus.
Gaussian Wave Packet:
- A Gaussian wave packet representing a particle with a well-defined position and momentum has the wave function Ψ(x,0)=
  $\left( \frac{1}{2 \pi \sigma^2} \right)^{1/4} \exp \left( -\frac{x^2}{4 \sigma^2} \right)$
- The probability density ∣Ψ(x,0)∣² is a Gaussian function centered at x=0x = 0x=0 with width σ\sigmaσ.

Practice Test Questions on Wave Function and Probability

Question 1

Which of the following expressions represents the probability density of finding a particle at position x for a given wave function Ψ(x,t)?

A. Ψ(x,t)

B. ∣Ψ(x,t)∣²

$C. \frac{\partial \Psi(x, t)}{\partial t}$

$\int_{-\infty}^{x} \Psi(x', t) \, dx'$

Answer: B. ∣Ψ(x,t)∣²

Explanation: The probability density ∣Ψ(x,t)∣2|\Psi(x, t)|^2∣Ψ(x,t)∣2 represents the likelihood of finding a particle at position xxx at time ttt. This is derived from the wave function Ψ(x,t)\Psi(x, t)Ψ(x,t), and by taking the square of its absolute value, we get the probability density.

Question 2:

What does the square of the absolute value of the wave function represent?

A) The momentum of the particle

B) The probability density of finding the particle at a given position

C) The total energy of the system

D) The wavelength of the particle

Answer: B) The probability density of finding the particle at a given position

Explanation: The square of the absolute value of the wave function represents the probability density, which gives the likelihood of finding the particle at a specific position in space at a given time.

Question 3:

Which principle states that certain pairs of physical properties, like position and momentum, cannot both be known to arbitrary precision?

A) Schrödinger’s Principle

B) Heisenberg Uncertainty Principle

C) Pauli Exclusion Principle

D) Conservation of Energy Principle

Answer: B) Heisenberg Uncertainty Principle

Explanation: The Heisenberg Uncertainty Principle states that it is impossible to simultaneously know both the exact position and exact momentum of a particle with arbitrary precision. This principle highlights the fundamental limits of measurements in quantum mechanics.