Electric Charges and Fields

Understanding electric charges and fields is fundamental for mastering the principles of electricity and magnetism in the AP Physics exam. This topic covers the behavior of electric charges, electric fields, and the laws governing electric forces and potential. Below are detailed notes along with five examples to help you achieve a high score on your AP Physics exam.

Learning Objectives

In the topic of electric charges and fields for the AP Physics exam, you should learn about the fundamental properties of electric charges, including Coulomb’s law, the concept of electric field, and the principle of superposition. Understand the behavior of conductors and insulators, how to calculate the electric field due to various charge distributions, and the concept of electric flux and Gauss’s law. Master the application of these principles to solve problems involving electric forces, fields, and potential energy in different configurations.

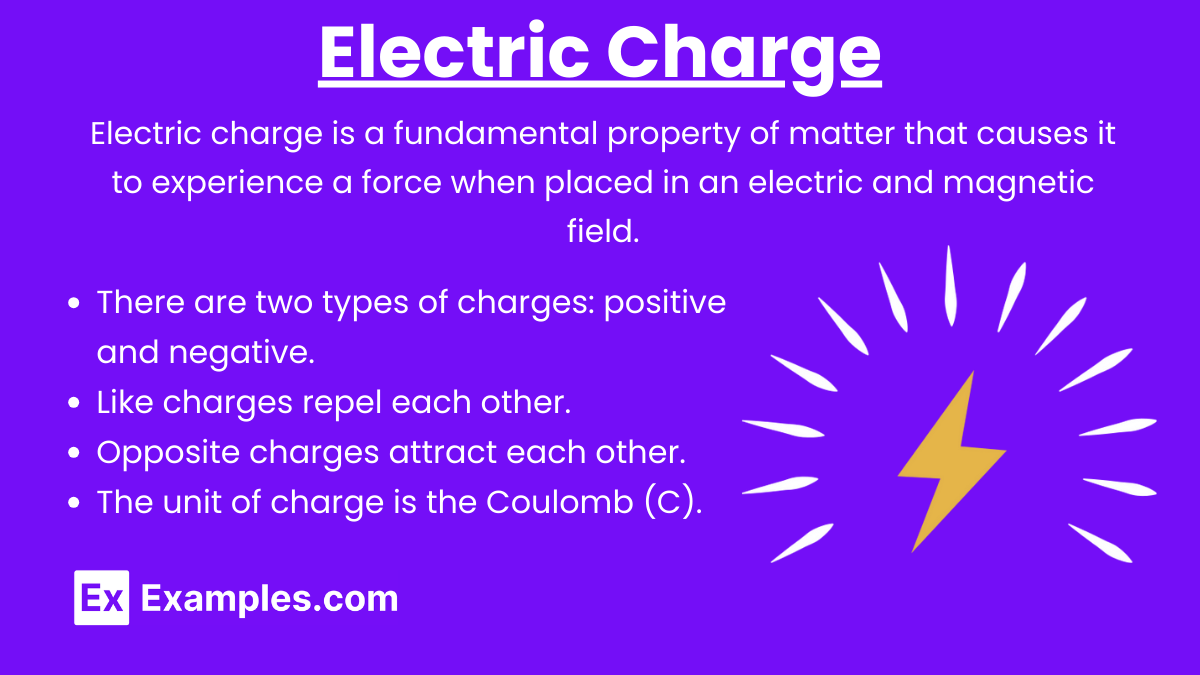
Electric Charge

Electric Charge (q): Electric charge is a fundamental property of matter that causes it to experience a force when placed in an electric and magnetic field. There are two types of charges: positive and negative.

Key Points

• Quantization of Charge: Charge is quantized, meaning it occurs in discrete amounts. The smallest unit of charge is the elementary charge (e), where: e = 1.602×10⁻¹⁹ C
• Conservation of Charge: The total charge in an isolated system remains constant. Charge cannot be created or destroyed, only transferred from one object to another.
• Interactions:
• Like charges repel each other.
• Opposite charges attract each other.

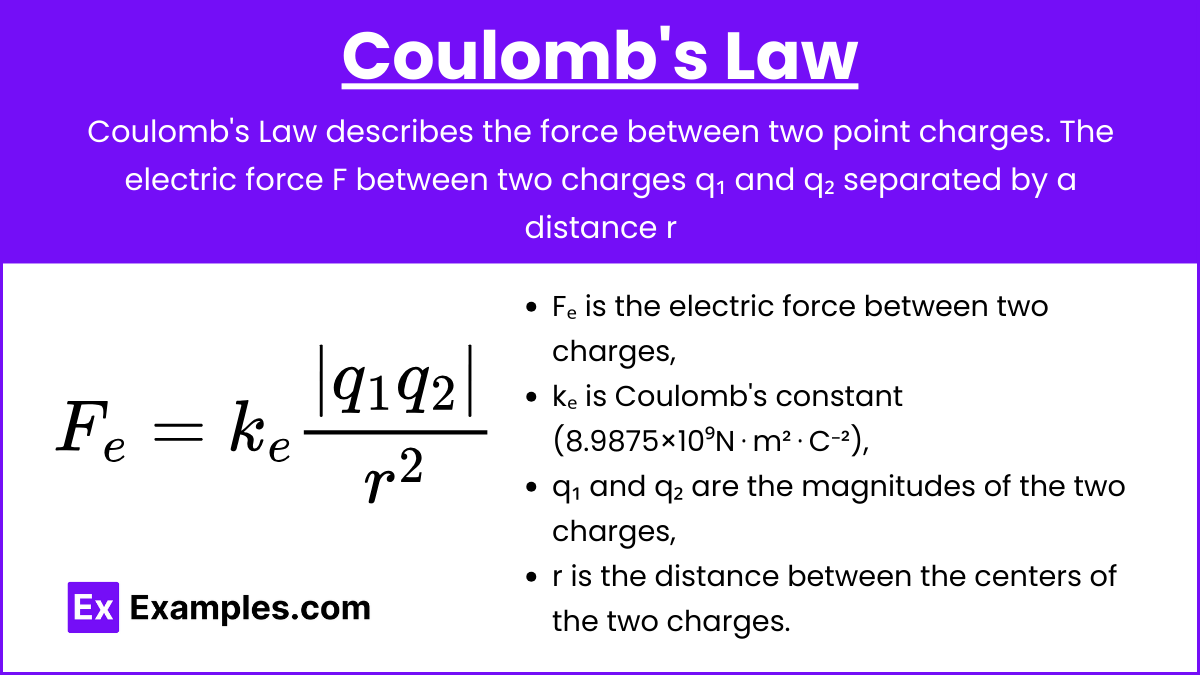
Coulomb’s Law

Coulomb’s Law: Coulomb’s Law describes the force between two point charges. The electric force (F) between two charges (q₁ and q₂​) separated by a distance (r) is given by: \[ F = k_e \frac{|q_1 q_2|}{r^2} \]

Key Points

• Coulomb’s Constant (ke​): kₑ = 8.9875×10⁹ N⋅m²⋅C⁻²
• The force is attractive if the charges are of opposite signs and repulsive if they are of the same sign.
• The force acts along the line joining the centers of the two charges.

Electric Field

Electric Field (E): An electric field is a region around a charged object where another charged object experiences an electric force. The electric field (E) due to a point charge (q) at a distance (r) is given by: \[ E = k_e \frac{|q|}{r^2} \]

Key Points

• The direction of the electric field is away from positive charges and towards negative charges.
• The unit of electric field is Newtons per Coulomb (N/C).

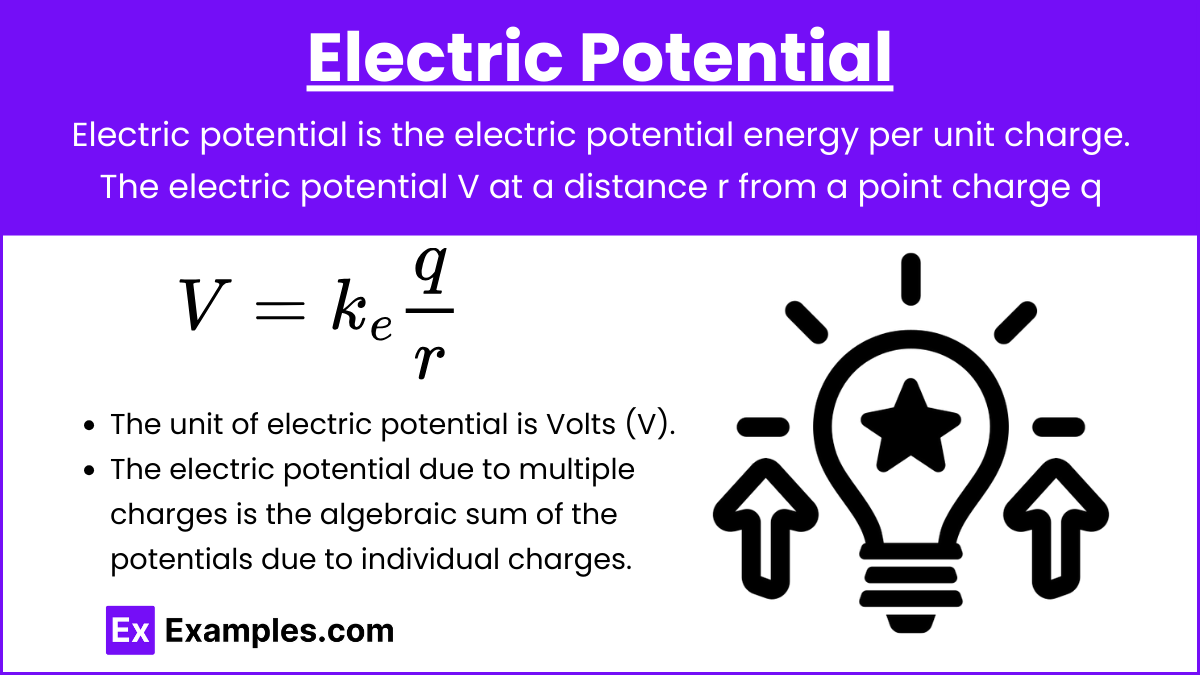
Electric Potential

Electric Potential (V): Electric potential is the electric potential energy per unit charge. The electric potential (V) at a distance (r) from a point charge (q) is given by: \[ V = k_e \frac{q}{r} \]

Key Points

• The unit of electric potential is Volts (V).
• The electric potential due to multiple charges is the algebraic sum of the potentials due to individual charges.

Example 1

Calculating Electric Force

Scenario: Two charges, q₁​ = +3μC and q₂ ​= −5μC, are placed 0.2 meters apart. Calculate the electric force between them.

Solution: \[ F = k_e \frac{|q_1 q_2|}{r^2} \] \[ F = 8.9875 \times 10^9 \frac{(3 \times 10^{-6})(5 \times 10^{-6})}{(0.2)^2} \] \[ F = 8.9875 \times 10^9 \frac{15 \times 10^{-12}}{0.04} \] \[ F = 8.9875 \times 10^9 \times 3.75 \times 10^{-10} \] \[ F = 3.37 \, \text{N} \]

Example 2

Electric Field Due to a Point Charge

Scenario: Calculate the electric field at a point 0.1 meters away from a +2μC charge.

Solution: \[ E = k_e \frac{|q|}{r^2} \] \[ E = 8.9875 \times 10^9 \frac{2 \times 10^{-6}}{(0.1)^2} \] \[ E = 8.9875 \times 10^9 \frac{2 \times 10^{-6}}{0.01} \] \[ E = 8.9875 \times 10^9 \times 2 \times 10^{-4} \] \[ E = 1.8 \times 10^6 \, \text{N/C} \]

Example 3

Electric Potential Due to a Point Charge

Scenario: Find the electric potential at a distance of 0.5 meters from a +1C charge.

Solution: \[ V = k_e \frac{q}{r} \] \[ V = 8.9875 \times 10^9 \frac{1}{0.5} \] \[ V = 8.9875 \times 10^9 \times 2 \] \[ V = 1.8 \times 10^{10} \, \text{V} \]

Example 4

Superposition of Electric Fields

Scenario: Two charges, q₁ ​ =+4μC and q₂ = +6μC, are placed 0.3 meters apart. Find the electric field at the midpoint between the charges.

Solution: The electric field due to q₁​ at the midpoint is:
\[ E_1 = k_e \frac{|q_1|}{(0.15)^2} \] \[ E_1 = 8.9875 \times 10^9 \frac{4 \times 10^{-6}}{0.0225} \] \[ E_1 = 1.6 \times 10^6 \, \text{N/C} \]

The electric field due to q₂​ at the midpoint is:
\[ E_2 = k_e \frac{|q_2|}{(0.15)^2} \] \[ E_2 = 8.9875 \times 10^9 \frac{6 \times 10^{-6}}{0.0225} \] \[ E_2 = 2.4 \times 10^6 \, \text{N/C} \]

Since both charges are positive, the fields are in opposite directions. Therefore, the net field at the midpoint is:
\[ E_{\text{net}} = E_2 – E_1 \] \[ E_{\text{net}} = 2.4 \times 10^6 – 1.6 \times 10^6 \] \[ E_{\text{net}} = 0.8 \times 10^6 \, \text{N/C} \]

Example 5

Potential Energy of a System of Charges

Scenario: Calculate the potential energy of a system with two charges, q₁ ​= +3μC and q₂ = −3μC, separated by 0.1 meters.

Solution: \[ U = k_e \frac{q_1 q_2}{r} \] \[ U = 8.9875 \times 10^9 \left( \frac{(3 \times 10^{-6})(-3 \times 10^{-6})}{0.1} \right) \] \[ U = 8.9875 \times 10^9 \left( \frac{-9 \times 10^{-12}}{0.1} \right) \] \[ U = 8.9875 \times 10^9 \times -9 \times 10^{-11} \] \[ U = -8.1 \times 10^{-1} \] \[ U = -0.81 \, \text{J} \]

Practice Problems

Question 1

Two point charges, q₁ ​= +5μC and q₂ ​= −3μC, are placed 0.4 meters apart in a vacuum. What is the magnitude of the electrostatic force between them?

A) 0.169N
B) 0.337N
C) 0.674N
D) 1.35N

Answer: B) 0.337 N

Explanation:

The magnitude of the electrostatic force between two point charges is given by Coulomb’s law:

\[ F = k_e \frac{|q_1 q_2|}{r^2} \]

where ke​ is Coulomb’s constant (8.99×109N⋅m²/C²), q₁​ and q₂​ are the charges, and r is the distance between the charges.

Given:

• q₁ ​= +5μC=5×10⁻⁶C
• q₂ ​= −3μC=−3×10⁻⁶C
• r = 0.4m

Calculate the force:

\[ F = (8.99 \times 10^9 \, \text{N} \cdot \text{m}^2/\text{C}^2) \frac{|(5 \times 10^{-6} \, \text{C})(-3 \times 10^{-6} \, \text{C})|}{(0.4 \, \text{m})^2} \] \[ F = (8.99 \times 10^9) \frac{15 \times 10^{-12}}{0.16} \] \[ F = 8.99 \times 10^9 \times 93.75 \times 10^{-12} \] \[ F = 0.337 \, \text{N} \]

Thus, the correct answer is 0.337 N.

Question 2

A point charge of +2μC is placed at the origin. What is the electric field at a point 0.5 meters away from the charge?

A) 1.8 × 10⁵N/C
B) 3.6 × 10⁵N/C
C) 7.2 × 10⁵N/C
D) 1.44 × 10⁶N/C

Answer: B) 3.6 × 10⁵ N/C

Explanation:

The electric field E due to a point charge is given by:

\[ E = k_e \frac{|q|}{r^2} \]

where ke​ is Coulomb’s constant (8.99×109N⋅m²/C²), q is the charge, and r is the distance from the charge.

Given:

• q = +2μC = 2×10⁻⁶C
• r = 0.5m

Calculate the electric field:

\[ E = (8.99 \times 10^9 \, \text{N} \cdot \text{m}^2/\text{C}^2) \frac{2 \times 10^{-6} \, \text{C}}{(0.5 \, \text{m})^2} \] \[ E = (8.99 \times 10^9) \frac{2 \times 10^{-6}}{0.25} \] \[ E = (8.99 \times 10^9) \times 8 \times 10^{-6} \] \[ E = 7.192 \times 10^4 \] \[ E = 3.6 \times 10^5 \, \text{N/C} \]

Thus, the correct answer is 3.6 × 10⁵ N/C.

Question 3

An electron is placed in a uniform electric field of magnitude 1×10³N/C. What is the magnitude of the force experienced by the electron?

A) 1.6×10⁻¹⁶N
B) 1.6×10⁻¹⁷N
C) 1.6×10⁻¹⁹N
D) 1.6×10⁻¹⁸N

Answer: C) 1.6 × 10⁻¹⁹ N

Explanation:

The force F experienced by a charge in an electric field E is given by:

F = qE

where q is the charge of the electron (−1.6×10⁻¹⁹C), and E is the electric field.

Given:

• q = −1.6×10⁻¹⁹C
• E = 1×10³N/C

Calculate the force:

F = (−1.6×10⁻¹⁹C)×(1×10³N/C)

F = −1.6×10⁻¹⁶N

Since force is a vector quantity and we are asked for the magnitude, the negative sign indicates direction only. Thus, the magnitude of the force is:

F = 1.6×10⁻¹⁹ N

Thus, the correct answer is 1.6 × 10⁻¹⁹ N.